This article originally appeared on the Mozilla Hacks blog.
That shopping rabbit hole you started on your laptop this morning? Pick up where you left off on your phone tonight. That dinner recipe you discovered at lunchtime? Open it on your kitchen tablet, instantly. Connect your personal devices, securely. – Firefox Sync
Firefox Sync lets you share your bookmarks, browsing history, passwords and other browser data between different devices, and send tabs from one device to another. It’s a feature that millions of our users take advantage of to streamline their lives and how they interact with the web.
But on an Internet where sharing your data with a provider is the norm, we think it’s important to highlight the privacy aspects of Firefox Sync.
Firefox Sync by default protects all your synced data so Mozilla can’t read it. We built Sync this way because we put user privacy first. In this post, we take a closer look at some of the technical design choices we made and why.
When building a browser and implementing a sync service, we think it’s important to look at what one might call ‘Total Cost of Ownership’. Not just what users get from a feature, but what they give up in exchange for ease of use.
We believe that by making the right choices to protect your privacy, we’ve also lowered the barrier to trying out Sync. When you sign up and choose a strong passphrase, your data is protected from both attackers and from Mozilla, so you can try out Sync without worry. Give it a shot, it’s right up there in the menu bar!
Why Firefox Sync is safe
Encryption allows one to protect data so that it is entirely unreadable without the key used to encrypt it. The math behind encryption is strong, has been tested for decades, and every government in the world uses it to protect its most valuable secrets.
The hard part of encryption is that key. What key do you encrypt with, where does it come from, where is it stored, and how does it move between places? Lots of cloud providers claim they encrypt your data, and they do. But they also have the key! While the encryption is not meaningless, it is a small measure, and does not protect the data against the most concerning threats.
The encryption key is the essential element. The service provider must never receive it – even temporarily – and must never know it. When you sign into your Firefox Account, you enter a username and passphrase, which are sent to the server. How is it that we can claim to never know your encryption key if that’s all you ever provide us? The difference is in how we handle your passphrase.
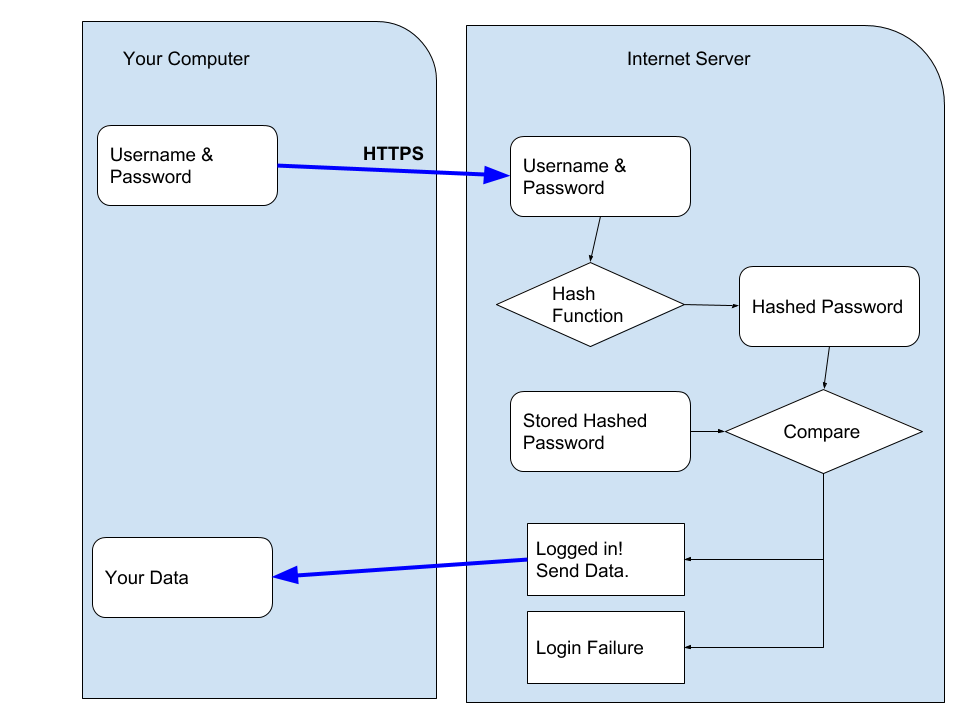
A typical login flow for an internet service is to send your username and passphrase up to the server, where they hash it, compare it to a stored hash, and if correct, the server sends you your data. (Hashing refers to the activity of converting passwords into unreadable strings of characters impossible to revert.)
The crux of the difference in how we designed Firefox Accounts, and Firefox Sync (our underlying syncing service), is that you never send us your passphrase. We transform your passphrase on your computer into two different, unrelated values. With one value, you cannot derive the other0. We send an authentication token, derived from your passphrase, to the server as the password-equivalent. And the encryption key derived from your passphrase never leaves your computer.
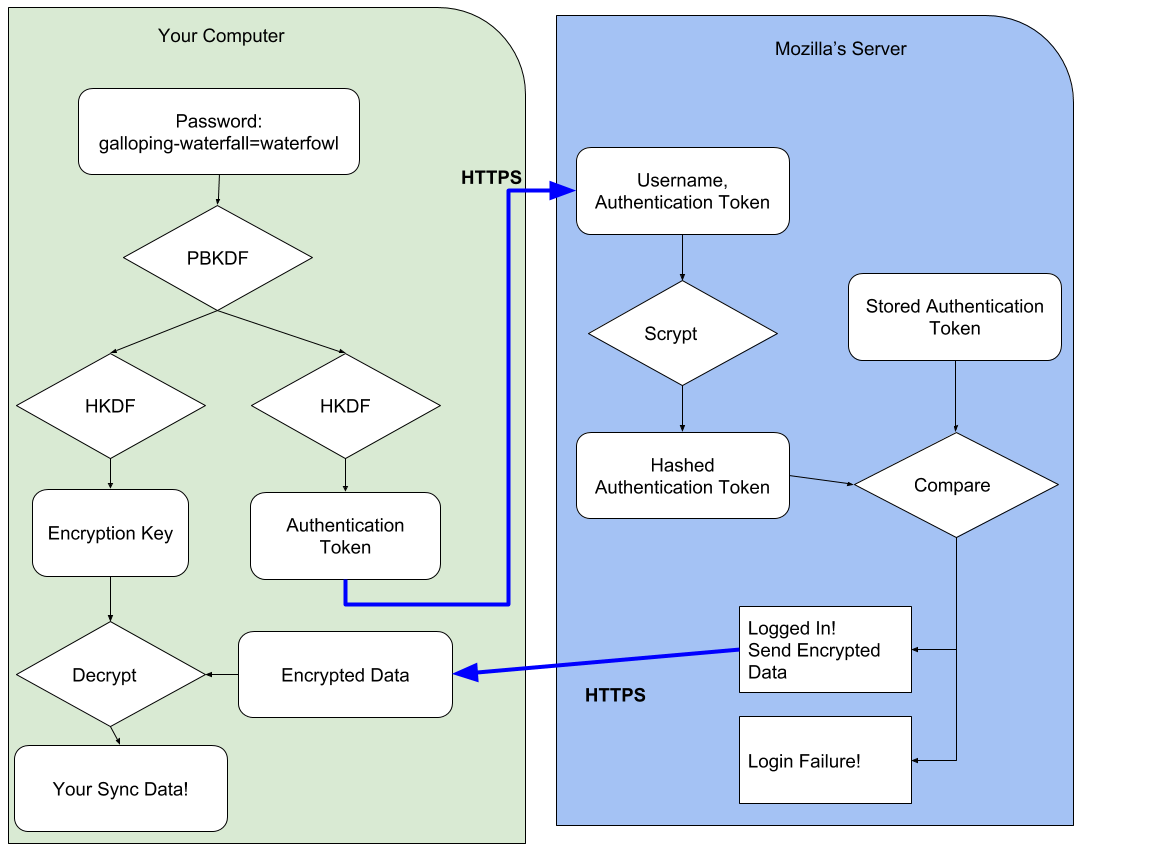
Interested in the technical details? We use 1000 rounds of PBKDF2 to derive your passphrase into the authentication token1. On the server, we additionally hash this token with scrypt (parameters N=65536, r=8, p=1)2 to make sure our database of authentication tokens is even more difficult to crack.
We derive your passphrase into an encryption key using the same 1000 rounds of PBKDF2. It is domain-separated from your authentication token by using HKDF with separate info values. We use this key to unwrap an encryption key (which you generated during setup and which we never see unwrapped), and that encryption key is used to protect your data. We use the key to encrypt your data using AES-256 in CBC mode, protected with an HMAC3.
This cryptographic design is solid – but the constants need to be updated. One thousand rounds of PBKDF can be improved, and we intend to do so in the future (Bug 1320222). This token is only ever sent over a HTTPS connection (with preloaded HPKP pins) and is not stored, so when we initially developed this and needed to support low-power, low-resources devices, a trade-off was made. AES-CBC + HMAC is acceptable – it would be nice to upgrade this to an authenticated mode sometime in the future.
Other approaches
This isn’t the only approach to building a browser sync feature. There are at least three other options:
Option 1: Share your data with the browser maker
In this approach, the browser maker is able to read your data, and use it to provide services to you. For example, when you sync your browser history in Chrome it will automatically go into your Web & App Activity unless you’ve changed the default settings. As Google Chrome Help explains, “Your activity may be used to personalize your experience on other Google products, like Search or ads. For example, you may see a news story recommended in your feed based on your Chrome history.”4
Option 2: Use a separate password for sign-in and encryption
We developed Firefox Sync to be as easy to use as possible, so we designed it from the ground up to derive an authentication token and an encryption key – and we never see the passphrase or the encryption key. One cannot safely derive an encryption key from a passphrase if the passphrase is sent to the server.
One could, however, add a second passphrase that is never sent to the server, and encrypt the data using that. Chrome provides this as a non-default option5. You can sign in to sync with your Google Account credentials; but you choose a separate passphrase to encrypt your data. It’s imperative you choose a separate passphrase though.
All-in-all, we don’t care for the design that requires a second passphrase. This approach is confusing to users. It’s very easy to choose the same (or similar) passphrase and negate the security of the design. It’s hard to determine which is more confusing: to require a second passphrase or to make it optional! Making it optional means it will be used very rarely. We don’t believe users should have to opt-in to privacy.
Option 3: Manual key synchronization
The key (pun intended) to auditing a cryptographic design is to ask about the key: “Where does it come from? Where does it go?” With the Firefox Sync design, you enter a passphrase of your choosing and it is used to derive an encryption key that never leaves your computer.
Another option for Sync is to remove user choice, and provide a passphrase for you (that never leaves your computer). This passphrase would be secure and unguessable – which is an advantage, but it would be near-impossible to remember – which is a disadvantage.
When you want to add a new device to sync to, you’d need your existing device nearby in order to manually read and type the passphrase into the new device. (You could also scan a QR code if your new device has a camera).
Other Browsers
Overall, Sync works the way it does because we feel it’s the best design choice. Options 1 and 2 don’t provide thorough user privacy protections by default. Option 3 results in lower user adoption and thus reduces the number of people we can help (more on this below).
As noted above, Chrome implements Option 1 by default, which means unless you change the settings before you enable sync, Google will see all of your browsing history and other data, and use it to market services to you. Chrome also implements Option 2 as an opt-in feature.
Opera and Vivaldi follow Chrome’s lead, implementing Option 1 by default and Option 2 as an opt-in feature. Update: Vivaldi actually prompts you for a separate password by default (Option 2), and allows you to opt-out and use your login password (Option 1).
Brave, also a privacy-focused browser, has implemented Option 3. And, in fact, Firefox also implemented a form of Option 3 in its original Sync Protocol, but we changed our design in April 2014 (Firefox 29) in response to user feedback6. For example, our original design (and Brave’s current design) makes it much harder to regain access to your data if you lose your device or it gets stolen. Passwords or passphrases make that experience substantially easier for the average user, and significantly increased Sync adoption by users.
Brave’s sync protocol has some interesting wrinkles7. One distinct minus is that you can’t change your passphrase, if it were to be stolen by malware. Another interesting wrinkle is that Brave does not keep track of how many or what types of devices you have. This is a nuanced security trade-off: having less information about the user is always desirable… The downside is that Brave can’t allow you to detect when a new device begins receiving your sync data or allow you to deauthorize it. We respect Brave’s decision. In Firefox, however, we have chosen to provide this additional security feature for users (at the cost of knowing more about their devices).
Conclusion
We designed Firefox Sync to protect your data – by default – so Mozilla can’t read it. We built it this way – despite trade-offs that make development and offering features more difficult – because we put user privacy first. At Mozilla, this priority is a core part of our mission to “ensure the Internet is a global public resource… where individuals can shape their own experience and are empowered, safe and independent.”
0 It is possible to use one to guess the other, but only if you choose a weak password. ⬑
1 You can find more details in the full protocol specification or by following the code starting at this point. There are a few details we have omitted to simplify this blog post, including the difference between kA and kB keys, and application-specific subkeys. ⬑
2 Server hashing code is located here. ⬑
3 The encryption code can be seen here. ⬑
4 https://support.google.com/chrome/answer/165139 Section “Use your Chrome history to personalize Google” ⬑
5 Chrome 71 says “For added security, Google Chrome will encrypt your data” and describes these two options as “Encrypt synced passwords with your Google username and password” and “Encrypt synced data with your own sync passphrase”. Despite this wording, only the sync passphrase option protects your data from Google. ⬑
6 One of the original engineers of Sync has written two blog posts about the transition to the new sync protocol, and why we did it. If you’re interested in the usability aspects of cryptography, we highly recommend you read them to see what we learned. ⬑
7 You can read more about Brave sync on Brave’s Design page. ⬑
Over time, I've accumulated a lot of ideas that I would love to work on myself, but have to admit I pretty much never will (there's only so many hours in the day.) At the same time, I regularly see project proposals (as part of the Advisory Councils for OTF and CII) that... while not bad, often don't inspire excitement in me. So I thought I'd write down some of my ideas in the hope that they inspire someone else.
Of note: I don't know about everything on the Internet. It's a certainty that someone really this uses something that I want on the daily. Please, leave a comment and point to implementations!
Ideas
Secure Mobile Encryption with a PIN
Why do you think the FBI had to go to Apple to unlock a suspect's iPhone; but they've never had to go to Google? On a new iPhone (emphasis new, older models don't apply), the '10 incorrect PINs erase the phone' functionality is backed by hardware and very difficult to bypass. On Android... there is such a landscape of phones that even if one of them had hardware backed security for the PIN (and I don't even know if one does!) you'd have to go out of your way to purchase that individual phone.
Now let's switch to the perspective of app developers. You want to build your app so if someone seizes or steals the user's phone, there's protection against brute force attacks trying to obtain a user's data. But with the landscape of Android being what it is, you can't rely on the lockscreen. (And recommending a user go buy a new phone is out of the question.) So as a developer you have to build the feature yourself. So if you encrypt the database, you have to assume the (encrypted) database can be extracted from the phone. There's no safe place to store the key on the phone so the only thing protecting against brute force is the user's PIN or password. And it's not like typing in a 10 word pass-poem is friendly on a phone - especially if it's required every time you open the app!
So as an application developer - you're screwed. There's no way to enable a user to have a good experience with your app and protect their data. But it doesn't have to be this way. An Android phone has a plethora of secure elements on it - hardware devices that are difficult for attackers to bypass. And the most universal one is... the SIM card.
Imagine an Android app that loads a small JavaCard applet onto the SIM Card. Upon app startup, the user creates a 4-digit PIN that is passed to and stored in the JavaCard applet. The JavaCard applet generates a random encryption key and passes it to the Android app, which uses it to encrypt that database that is stored on the phone. Next time you start up the Android app, you enter a PIN - which gets passed to the JavaCard applet. If the PIN matches what's stored in the applet, the applet returns the encryption key and the app uses it to decrypt the database. But after 10 wrong tries the applet erases the key - locking the attacker out of the database forever. The important point here is that the PIN (or encryption key) is difficult to extract from the SIM card because that's why SIM cards exist - to have a small secure element where it's difficult to steal data from.
Just like that, we have enforceable brute force protection for even 4 digit PINs. What to build this? Where do you get started? Well, SEEK for Android is an Android patch that adds a SmartCard API. Nikolay Elenkov wrote a blog post several years ago about doing something very similar to this idea.
Regrettably the end-game for this is somewhat limited. It's impossible to load JavaCard applets onto normal US carrier SIMs (because they're locked down). You can buy pretty nice Dual-SIM Android phones and put a carrier SIM in one slot and a programmable SIM in the other slot. But this doesn't solve the 'Don't require people to buy a new phone' problem. This does seem like the type of thing that Copperhead would be interested in (and Lineageos and potentially other Android OSes).
Privacy Preserving Location Sharing
Location is a pretty personal thing. No one wants to give their location to some third party to store forever and track us. Nor does anyone want to constantly give out their location to a huge list of friends or acquaintances on the off chance one might be 'nearby'. But when you're meeting someone, or traveling to a new city, or going out to the bar with friends, or a host of other scenarios - it would be nice to share your location automatically. An app that shares location, with a lot of privacy settings and geo-fences, sounds like a really useful tool. Could it exist?
It could! A paper was published talking about how to accomplish it in 2007. Since then it's been cited something like 170 times which implies there might have been some improvements. In 2008 this was implemented as NearbyFriend; and in 2012 it was updated (kinda) to use a more free geolocation API. But both projects have sat dormant.
I think that's a shame, and more than a shame - it's an opportunity. This functionality sits well with the end-to-end encrypted messengers we use daily. Some of the features I would want would include controlling location granularity, geo-fences around places I don't ever want to 'be', and 'muting' contacts so that they can't tell I'm purposely not sharing my location with them.
Remote Server Attestation of OSS Server Configs
When it comes to UEFI and Secure Boot and all that jazz, I kind of wave my hands around and butcher what Ted Reed has told me in various bars and airports. So without adieu... /me begins waving his hands.
Secure Boot is this term for saying that when your computer boots up, it does so (or can do so if you chant the right incantations) into a kernel that is signed. The entire boot process moves from a signed BIOS to a signed kernel and signed kernel modules. We want to take this a step further with Remote Attestation. Remote Attestation is a way of saying "This is the hash of all the code I am running currently." That includes the kernel and can include userspace. The hash is signed by a key that is baked into hardware.
Remote Attestation got a bad rap because one of its initial goals was to ensure you weren't bypassing DRM, and because it generally had no provisions for privacy protection (that key, after all, is a baked-in permanent identifier you couldn't change.) But instead of using it on individuals laptops, let's turn it around and use it on servers. It would be great to enable some transparency into what sorts of things are happening on service providers' servers and there's plenty of open source projects who handle user data that I'm sure would like to provide even greater transparency to their operations. So, set up your servers using Docker or Puppet or whatever and publish exactly what you are running on them, and allow the general public to use Remote Attestation to confirm that the server has not been modified from that configuration in any way. (It would also enable the service provider themselves to know if their servers were tampered with!)
This is hardly a weekend project. Secure Boot itself is finicky and that's not even getting into Remote Attestation. And there will be bypasses - both of Secure Boot and the integrity of the system that is being attested. But with each bypass we can (hopefully) improve the system and finally reach the goal of being able to verify, remotely, the integrity and transparency of a running server.
Open Source TPM-Backed, Authenticated Disk Crypto
I was pretty heavily involved in the TrueCrypt audit and I've played with BitLocker too. I'm not a huge fan of either of them. Here's what I want in disk encryption software:
- Open Source - like TrueCrypt
- Backed by a TPM - like BitLocker
- Multiplatform - like TrueCrypt
- Supports Full-Disk or Containers/Volumes - like TrueCrypt
- Doesn't use XTS mode. At this point I think I would be happy with either Elephant from BitLocker, or an Authenticated Encryption mode like... no one. (I'd be fine with it eating up some of my disk space and performance storing authentication tags and doing a three phase commit (write new tag, write data, overwrite old tag))
The whole 'hidden container' / 'hidden operating system' notion is... really cool. But I've never examined how easy or difficult it is to detect them in a realistic setting. And I am extremely skeptical even knowledgeable users have the discipline needed to maintain the 'cover volume' in a way that appears convincing to the authorities. So this would be neat but far from required.
There are other features that'd be nice for power users or enterprise customers, sure. Additional key slots for enterprise decryption; removable bootloader like luks on Linux. But they're not the standard feature set needed by the average person.
Authenticated WebRTC Video Chats
In the beginning (well not really, but I'm going to play fast and loose with how I refer to 'time' for this section) there was RedPhone and Silent Circle and we had this ZRTP thing and we could do encrypted calls on our phones and it seemed great. And then Skype and Facetime and Google Hangouts and Facebook Chat and the like came along (well they were already there but pretend with me) and they had video calls. And here we were with our (admittedly crappy) encrypted audio.
But it doesn't have to be this way. Why don't we have open source, end-to-end encrypted video chat? WebRTC is built into open source browsers!
If you've never looked at WebRTC I don't blame you. But let you tell you a few things about it. WebRTC usually uses a STUN server to coordinate a peer-to-peer connection, but if the p2p connection fails, a TURN server can be used to pass the (encrypted) media stream back and forth. The media stream is encrypted using DTLS. Now if the other side of your DTLS connection is just a user with a web browser, what certificate are they using and how would you validate it? The answer is: a random certificate and you disable validation. But the important point is that WebRTC exposes the certificate.
So if we had some sort of end-to-end encrypted and authenticated chat, we could use that to bootstrap verification of WebRTC certificates! (...looks around optimistically...) Of course that's only part of the work, you would also need to go and find some open source self-hosted RTC setup to build upon...
Mic and Audio Cutoffs
The first two laptops I owned (that weren't my father's) had a toggle switch on the side to turn the WiFi on and off. I don't know how they worked - if it was a software switch or a power switch. But in a world where even the pope covers his camera with a sticker it's high time laptops came with a hard power switch for the camera and microphone. And I don't mean a software switch (we've seen too many examples of those being bypassed) I mean an honest to god 'If this LED is not lit then the microphone and camera are not getting power' switch.
It would be super-keen to have some kickstarter create usb and audio jack shims that add this feature too, so you can retrofit existing desktop-like setups, but this seems like too much of a niche market since most users could either unplug the accessories or have them built in and unremovable.
It was pointed out to me that Purism makes laptops with this feature!
Encrypted Broadcast Pager Network for SMS
You know what has the potential to be surprisingly private? Pagers. I'm not going to pretend I know anything about Pagers historically or how they're implemented today - but I do know that encrypted broadcast networks can be secure. Imagine one-way pagers, with an encryption key baked into a SIM card, coupled with local or satellite transmitters. You're going to need to compromise on this like Forward Secrecy, but with products like goTenna and beartooth - antiquated technology is getting a new lease on life with applied correctly. I have to wonder if this would be helpful in places with unreliable or unsafe internet.
More Better Faster Compiler Hardening
Exploit Mitigations like Control Flow Integrity are great. What's not great is the performance loss. Don't get me wrong, it's gotten leaps and bounds better over the years but the truth of the matter is - that performance cost still holds back organizations from deploying the hardening features. So anything that can be done to make Control Flow Integrity, Memory Partitioning, Virtual Table Verification, or similar features faster gets my enthusiasm.
Oh and Microsoft, for christ-sakes, it's been five years let the rest of us use vtguard.
Update & Binary Transparency
We're getting some good experience with Certificate Transparency; and we're also starting to flesh out some notions of Gossip (and that it's hard and that maybe it won't work the way we thought, but we're finally starting to talk about it.) It's time to move to the next two items on the list: Update and Binary Transparency.
Let's tackle the easier one first: Update Transparency (or UT). Lots of applications, especially browsers, have small components in them that auto-update. Extensions, Safebrowsing lists, PKI revocation information, and the browser updates themselves. Each of these 'update packages' stands on its own as a discrete chunk of data. Why not require the (hash of the) data to be present in a Transparency Log, with a STH and inclusion proof before the update is accepted by the browser?
One would have to think through how Gossip might work for this. We'll assume that there are independent auditors that come configured with the application (a browser in this case) and/or can be added manually. When a browser receives an 'update package', before it applies it, it will send the STH to the auditors. This could be done a few ways:
- Over pinned HTTPS directly to the auditor. This reveals user identity and behavior to the auditor but enables confirmation that the auditor received and processed the STH.
- Using DNS. This obscures user identity (to the point of DNS resolver) but does not ensure to the application that the auditor received the data
- Over a proxied connection to the auditor, routed through the browser manufacturer. The browser establishes a secure connection to the browser manufacturer, then creates an inner secure connection to one or more auditors. Done correctly, this should obscure user identity, although like the other two it does reveal general usage information. I think this is probably the best option.
Update transparency, while not simple, is simpler than Binary Transparency. When trying to think through Binary Transparency you run into concerns like a package's dependencies, different compilation options, it requires reproducible builds to start with (which in turn requires a very rigid toolchain...) That's not to say it shouldn't be explored also, but I think the next application of append-only Merkle Trees should be Update Transparency.
Encrypted Email Delivery
Email, and email security, is kind of confusing. MUA, MSA, MTA, MDA, SMTP, SMTPS, POP, POPS, IMAP, IMAPS, StartSSL, and that's not even getting into SPF, DMARC, DKIM, DANE or (god forbid) encrypted email (of the PGP or S/MIME variety.) I'm just going to talk about normal email. Like you use.
Hopefully when you check your email (and it's not in a browser), you do so using either POP or IMAP either over TLS or using StartSSL. The certificate that's returned to you is actually valid. That is, if you're checking your gmail, and you try to connect to imap.gmail.com - you get a certificate valid for imap.gmail.com. When you send an email, you do so using SMTP over TLS or using StartSSL and, again, you get a valid certificate. If you don't get a valid certificate or you cannot perform a StartSSL upgrade from plaintext to TLS - the connection fails.
Now let me take an aside right here. This is what happens if you use gmail or email through your company etc etc. It's not what happens if you get your email from Earthlink or from Uncle Bob's Rural Internet Provider, Hanggliding, and BBQ. I know this, for a fact, because for Earthlink Robert Graham told me so and for the the latter I have family who get their Internet from Uncle Bob and TLS is not supported. Which means it's not just their email going over insecure connections, it's their passwords too. But don't worry I'm sure they don't reuse the password. (Heh.)
Okay, let's come back to it. After you send your email, it goes from your Mail User Agent (MUA) to a Mail Submission Agent (MSA) to a Mail Transfer Agent (MTA). (The MSA and MTA are usually combined though.) The MTA transfers the email to an MTA run by the email provider of the recipient (let's imagine someone on yahoo email someone on gmail.) This Yahoo-MTA to Gmail-MTA connection is the weak point in the chain. MTAs rarely have correct TLS certificates for them, but even if they did - it wouldn't help. You see you find the MTA by looking up the MX record from DNS, and DNS is insecure. So even if the MTA required a valid certificate, the attacker could forge an MX record that points to their domain, that they have a valid certificate for.
It gets worse. Some MTAs don't support TLS at all. Combined with the certificate problem, we have a three-step problem. Some MTAs don't support TLS, so no MTA can require TLS unless it refuses to talk to some of the internet. Many MTAs that have TLS don't have valid certificates, so no MTA can require valid certificates unless it refuses to talk to some of the internet. And even if it has a valid certificate, almost no one has deployed DNSSEC so no MTA can require DNSSEC unless it refuses to talk to almost the entire internet. Google publishes some data about this.
BUT! We have solutions to these problems. They're actively being worked on over in the IETF's UTA group. One draft is for pinning TLS support (and it has a complimentary error reporting draft.) To secure the MX records, there's DANE but it requires DNSSEC.
Implementing these drafts and working on these problems makes tangible impact to the security and privacy of millions of people.
Delay Tolerant Networking
DTN is one of those things that exists today, but you don't think about it or realize it. I mean NASA has to talk to Curiosity somehow! The IETF has gotten in on the game too. I'm not really going to lie to you - I don't know what's going on in the DTN space. But I know it has a lot of applications that I'm interested in.
- Mix Networks
- Mesh Networks, like those used in Taiwanese protects
- Spotty connections, such as satellite downlinks and modems. think of how Telecomix got some internet into and out of Egypt during the Arab Spring
- Offline networks, where data is transmitted via USB Drives - which is hugely popular in Cuba and, I believe, parts of Africa
- Peer-to-peer exchanges, like the one Guardian Project is developing
I'm kind of lumping disconnected peer-to-peer data exchange in with true 'delayed networking' but whatever. They're similar. Understanding how to build applications using what are hopefully safe and interoperable protocols sounds like an important path forward.
Email Labels
Come on world. It's 2017. Labels won, folders lost. Why is gmail the only real system out there using labels? I mean we have hardlinks in the filesystem for crying out loud.
There seems to be a thing called 'notmuch' that a few people I know use, and it might have labels (sorry 'tags')... But where's the webmail support? Thunderbird (RIP...)?
Encrypted Email Databases
You know what was really cool? Lavabit. I don't know about Lavabit today (they have been up to a whole lot of work lately which I should really investigate) but let's talk about Lavabit the day before the government demanded Snowden's emails.
Lavabit was designed so that the user's emails were encrypted with the user's email password. The password itself was sent to the server in plaintext (over TLS), so the server certainly could store the password and have complete access to the user's data - this is not groundbreaking impenetrable security. But you know what? It's completely transparent to the user, and it's better than leaving the emails laying around in plaintext.
Why haven't we made some subtle improvements to this design and deployed it? Why are we letting the perfect be the enemy of the good?
Intel SGX Applications
SGX allows you to take a bundle of code and lock it away inside a container where no one, not even root, can access its data or modify its code. It's both inspiring and terrifying. What amounts to giving every consumer their own HSM for free, and terrifying undebuggable malware. I can think of a lot of things one can do with this, including:
- Develop ports of gpg-agent and ssh-agent that move your key material into an SGX container
- Develop something similar for TLS keys in apache/nginx
- Create a SGX application that tries to detect other SGX applications running on the machine using side-channels such as allocating all memory reserved for SGX
- And if doing so can block other applications from running
- Investigate if EGETKEY can be used as a tracking vector. (See this tweet.)
- And creating an application that factory resets and rotates the SGX keys every day
grsecurity
grsecurity is pretty awesome. I would love for it to more integrated (more features upstreamed) and easier to use (an ubuntu distribution for example). I can't claim to be familiar with the attempts that have been made in the past, I can also dream of a future where the enhanced security it provides is easily available to all.
Apparently coldkernel is a project that makes it easier to use grsecurity, although it says it's "extremely alpha".
Encrypted DNS
Over in the DPRIVE group in the IETF folks are developing how exactly to put DNS into TLS and DTLS. It's not that difficult, and you wind up with a relatively straightforward, multiplexed protocol. (The multiplexed is absolutely critical for performance so I want to mention that up front.) But the problem with encrypted DNS isn't the protocol. It's the deployment.
Right now the majority of people get their DNS from one of two places: their ISP or Google. The purpose of encrypted DNS is to protect your DNS queries from local network tampering and sniffing. So who do you choose to be on the other end of your encrypted DNS tunnel? Assuming they roll out the feature, do you choose Google? Or do we hope our ISPs provide it? Who hosts the encrypted DNS is a pretty big problem in this ecosystem.
The second big problem in this ecosystem is how applications on your machine perform DNS queries. The answer to that is getaddrinfo or gethostbyname - functions provided by the Operating System. So the OS is really the first mover in this equation - it needs to build in support for encrypted DNS lookup. But nameservers are almost always obtained by DHCP leases, so we need to get the DHCP servers to send locations of (D)TLS-supporting DNS resolvers once we somehow convince ISPs they should run them.
There's one other option, besides the OS, that could build support for encrypted DNS, and that's the browser. A browser could build the feature and send all its DNS requests encrypted and that would make a difference to users.
But, if a browser was to ship this feature before the OS, they would need to set a default encrypted DNS server to use. Let's be very clear about what we're talking about here: the browser is adding a dependency on an external service, such that if the service stops working the browser either breaks or performance degrades. We know, because we have seen over and over again, that browsers will not (and reasonably can not) rely on some third party who's expected to maintain good enough uptime and latency to keep their product working. So this means the browser has no choice but to run the encrypted DNS server themselves, and thereby making their product phone home for every browsing interaction you make. And that's worse, to them, than sending the DNS in plaintext.
Magic Folder
Dropbox and all these cloud syncronized folders things sure seem great. But maaaaybe I don't really want to give them all my files in plaintext. Surely there's something the cryptography can do here, right? Either a Dropbox alternative that encrypts the files and stores them in a cloud, or a shim that encrypts the files in folder 'Plaintext' and puts the ciphertext into Dropbox's syncced folder. (And to be clear, I'm not interested in encrypted backup, I'm interested in encrypted file synchronization.)
There are a couple of contenders - there's sparkleshare which is basically a hopefully-friendly UI over a git repo that you can access over Tor if you wish. And the encrypted backup service TAHOE-LAFS is working on a Magic Folder feature also.
I also know there are a lot of commercial offerings out there - I would start here for researching both Dropbox alternatives and encrypting shims. But my hopes aren't too high since I want something cross-platform, open source, with a configurable cloud destination (either my own location or space I pay them for.)
Services Hosting
Google seems to be pretty decent when it comes to fighting for the user - they push back on legal requests that seem to be over-broad and don't just roll over when threatened. But who would you trust more to fight against legal requests: Google.... or the ACLU? Google... or the EFF?
I would pay a pretty penny to either the ACLU or EFF to host my email. Today, more and more services are being centralized and turning into de-facto monopolies. Google (email, dns, and often an ISP), Akamai and Cloudflare (internet traffic), Charter and Comcast (ISPs). It's surprisingly hard to get information about what portion of the internet is in Amazon EC2 - a 2012 report said 1% of the Internet (with one-third of internet users accessing it daily) and the Loudon, Virginia county economic-development board claimed in 2015/2016 that 70% of the internet's traffic worldwide goes through that region. This centralization of the internet into a few providers is happening in conjunction with the region-izing the Internet by promoting national services over international (China is most famous for this, but you see it elsewhere too.) And when national services can't compete, the government incentivizes companies like Google to set up offices and data centers - which seems like a no brainer until you realize the legal implications of pissing off a government who has jurisdiction of your employees and facilities.
The Internet was built to be decentralized and federated, and we're losing that. Furthermore, we're delegating more of our data to publicly traded third party companies - and with that data goes our rights. So I'd like to trust the people who are most invested in protecting our rights with my data - instead of the companies.
And there's a lot more than just email that I'd like them to run. I'd love for them to be my ISP for one. Then there's Google's DNS servers which see god-knows-how-much of the internet's traffic. I'm really uncomfortable with them getting that amount of data about my everyday web usage. There are good-of-the-internet public services like Certificate Transparency Logs and Auditors, the Encrypted DNS and Magic Folder services I mentioned earlier, as well as Tor nodes. But let's start with something simple: VPS Hosting and Email. Those are easily monetized.
RDNC & OMAPI
What the heck are these? RDNC is a remote administration tool for BIND. OMAPI is a remote administration tool for DHCPd. As far as I can tell, both protocols are obscure and rarely investigated and only meant to be exposed to authorized hosts. But back when I did network penetration tests they were always popping up. I never got the chance to fuzz or audit them, but I bet you money that there are cryptographic errors, memory corruption, and logic errors lurking inside these extremely popular daemons. Want to make the Internet upgrade? This is my secret I'm telling you - start here.
XMPP Chat Relay Network
Alright, this one is kind of out there. Stay with me through it. First - let's assume we want to keep (and invest in) federated XMPP even though there's some compelling reasons that isn't a great idea. So we've got XMPP (and OTR - pretend we got everyone OTR and upgraded the protocol to be more like Signal). There's some downsides here in terms of metadata - your server has your address book and it knows who you talk to, when, and the OTR ciphertext. Let's solve those problems.
First, let's create a volunteer, community run network of XMPP servers that allow anonymous registration. (Kind of like the Tor network, but they're running XMPP servers.) These servers auto-register to some directory, just like the Tor network, and your client downloads this directory periodically. They don't live forever, but they're reasonably long-lived (on the order of months) and have good uptime.
Second, let's create an incredibly sophisticated 'all-logic on the client' XMPP client. This XMPP client is going to act like a normal XMPP client that talks to your home server, but it also builds in OTR, retrieves that directory of semi-ephemeral servers, and does a whole lot more logic we will illustrate.
Let's watch what happens when Alice wants to talk to Bob. Alice creates several (we'll say three) completely ephemeral identities (maybe over Tor, maybe not) on three ephemeral servers, chooses one and starts a conversation with bob@example.com. There's an outer handshake, but subsequent to that, Alice identifies herself not only as jh43k45bdk@j4j56bdi4.com but also as her 'real identity' alice@example.net. Now that Bob knows who he's talking to, he replies 'Talk to me at qbhefeu4v@zbdkd3k5bf.com with key X' (which is one of three new ephemeral accounts he makes on ephemeral servers). Alice does so, changing the account she used in the process. Now Alice and Bob are talking through an ephemeral server who has no idea who's talking.
This type of protocol needs a lot of fleshing out, but the goals are that 'home' servers provide a friendly and persistent way for a stranger to locate a known contact but that they receive extremely little metadata. The XMPP servers that see ciphertext don't see identities. The XMPP servers that see identities don't see ciphertext. Clients regularly rotate ephemeral addresses to communicate with.
Mix Networks for HTTP/3
Here's another one that's pretty far out there. Look at your browser. Do you have facebook, twitter, or gmail open (or some other webmail or even outlook/thunderbird) - idling in some background tab, occasionally sending you notices or alerts? I'd be surprised if you didn't. A huge portion of the web uses these applications and a huge portion of their usage is sitting, idle, receiving status updates.
HTTP/2 was designed to be compatible with HTTP/1. Specifically, while the underlying transport changed to a multiplexed protocol with new compression applied - the notion of request-response with Headers and a Body remained unchanged. I doubt HTTP/3 will make any such compatibility efforts. Let's imagine what it might be then. Well, we can expect that in the future more and more people have high-bandwidth connections (we're seeing this move to fiber and gigabit now) but latency will still stink. Don't get me wrong, it will go down, but it's still slow comparatively. That's while there's the big push for protocols with fewer round trips. There's a lot of 'stuff' you have to download to use gmail, and even though now it's multiplexed and maybe even server push-ed the 'startup' time of gmail is still present. Gmail has a loading indicator. Facebook does too.
So I could imagine HTTP/3 taking the notion of server push even further. I can easily imagine you downloading some sort of 'pack'. A zipfile, an 'app', whatever you want to call it. It's got some index page or autorun file and the 'app' will load its logic, its style, its images, and maybe even some preloaded personal data all from this pack. Server push taken even further. Periodically, you'll receive updates - new emails will come in, new tweets, new status posts, new ads to display. You might even receive code updates (or just a brand-new pack that causes what we think of today as 'a page refresh' but might in the future be known as an 'app restart').
So if this is what HTTP/3 looks like... where does the Mix Network come in? Well... Mix Networks provide strong anonymity even in the face of a Global Passive Adversary, but they do this at the cost of speed. (You can read more about this over here and in these slides.) We'd like to use Mix Networks more but there's just no 'killer app' for them. You need something that can tolerate high latency. Recent designs for mix networks (and DC-nets) can cut the latency down quite a bit more from the 'hours to days' of ye olde remailers - but in all cases mix networks need to have enough users to provide a good anonymity set. And email worked as a Mix Network... kinda. You had reliability and spam problems, plus usability.
But what if HTTP/3 was the killer app that Mix Networks need. In Tor had a hybrid option - where some requests got mixed (causing higher latency) and others did not (for normal web browsing) - you could imagine a website that loaded it's 'pack' over onion routing, and then periodically sent you data updates using a mix network. If I'm leaving my browser sitting idle, and it takes the browser 5 or 10 minutes to alert me I have a new email instead of 1, I think I can live with that. (Especially if I can manually check for new messages.) I really should get less distracted by new emails anyway!
Sources of Funding
Okay, so maybe you like one of these ideas or maybe you think they're all shit but you have your own. You can do this. Required Disclaimer: While I am affiliated with some of these, I do not make the first or last funding decision for any of them and am speaking only for myself in this blog post. They may all think my ideas are horrible.
Open Tech Fund is a great place to bring whole application ideas (or improvements to applications) - stuff like the Privacy Preserving Location Sharing, Secure Mobile Encryption with a PIN, or Authenticated WebRTC Video Chats. Take those to their Internet Freedom Fund. You can also propose ideas to the Core Infrastructure Fund - Encrypted Email Delivery and Encrypted DNS implementations are great examples. In theory, they might take some of the way-far-out-there experiments (Update Transparency, Remote Server Attestation, Mix Networks) - but you'd probably need to put in some legwork first and really suss out your idea and make them believe it can be done.
The Linux Foundation's Core Infrastructure Initiative is really focusing on just that - Core Infrastructure. They tend to be interested in ideas that are very, very broadly applicable to the internet, which I have not focused on as much in this post. Better Faster Compiler Hardening is a good candidate, as is grsecurity (but that's a can of worms as I mentioned.) Still, if you can make a good case for how something is truly core infrastructure, you can try!
Mozilla's MOSS program has a track, Mission Partners that is the generic "Support projects that further Mozilla's mission" fund. It's the most applicable to the ideas here, although if you can make a good case that Mozilla relies on something you want to develop, you could make a case for the Foundational Technology fund (maybe compiler hardening or update transparency).
And there are a lot more (like a lot more) funding sources are out there. Not every source fits every idea of course, but if you want to unshackle yourself from a job doing things you don't care about and work on Liberation Technology - the options are more diverse than you might think.
Thanks
This was a big-ass blog post. Errors are my own, but Aaron Grattafiori and Drew Suarez helped fix many of them. Dan Blah pushed me to write it.
For the past year and change I've been working with dkg and Linus Nordberg on Certificate Transparency Gossip. I'll assume you're familiar with Certificate Transparency (you can read more about it here.) The point of CT Gossip is to detect Certificate Transparency logs that have misbehaved (either accidentally, maliciously, or by having been compromised.)
The CT Gossip spec is large, and complicated - perhaps too complicated to be fully implemented! This blog post is not about an overview of the specification, but rather about a nuanced problem we faced during the development - and why we made the decision we made. I'll take this problem largely into the abstract - focusing on the difficulty of providing protections against an intelligent adversary with statistics on their side. I won't reframe the problem or go back to the drawing board here. I imagine someone will want to, and we can have that debate. But right now I want to focus on the problem directly in front of us.
The Problem
In several points of the Gossip protocol an entity will have a bucket of items. We will call the entity the 'server' for simplicity - this is not always the case, but even when it is the web browser (a client), we can model it as a server. So the server has a bucket of items and a client (who will be our adversary) can request items from the bucket.
The server will respond with a selection of items of its choosing - which items and how many to respond with are choices the server makes. The server also chooses to delete items from the bucket at a time and by a policy of the server's choosing.
What's in the bucket? Well by and large they are innocuous items. But when an adversary performs an attack - evidence of that attack is placed into the bucket. The goal of the adversary is to 'flush' the evidence out of the bucket such that it is not sent to any legitimate clients, and is only sent to the adversary (who will of course delete the evidence of their attack.) Besides requesting items from the bucket, the attacker can place (innocuous) items into the bucket, causing the bucket to require more storage space.
The adversary can create any number of Sybils (or fake identities) - so there's no point in the server trying to track who they send an item to in an effort to send it to a diversity of requestors. We assume this approach will always fail, as the adversary can simply create false identities on different network segments.
Similarly, it's not clear how to distinguish normal client queries from an adversary performing a flushing attack. So we don't make an effort to do so.
Our goal is to define policies for the 'Release' Algorithm (aka 'which items from the bucket do I send') and the 'Deletion' Algorithm (aka 'do I delete this item from the bucket') such that an attacker is unsure about whether or not a particular item (evidence of their attack) actually remains in the bucket - or if they have successfully flushed it.
Published Literature
This problem is tantalizingly close to existing problems that exist in mix networks. Perhaps the best treatment of the flushing attack, and how different mixing algorithms resist it, is From a Trickle to a Flood from 2002.
But as intimated - while the problem is close, it is not the same. In particular, when (most | deployed) mix networks release a message, they remove it from the server. They do not retain it and send a duplicate of it later. Whereas in our situation, that is absolutely the case. This difference is very important.
The second difference is the attacker's goal. With Mix Networks, the attacker's goal is not to censor or discard messages, but instead to track them. In our model, we do want to eliminate messages from the network.
Defining The Attacker
So we have defined the problem: Server has a bucket. Attacker wants to flush an item from the bucket. How can we make the attacker unsure if they've flushed it? But we haven't defined the capabilities of the attacker.
To start with, we assume the attacker knows the algorithm. The server will draw random numbers during it, but the probabilities that actions will be taken are fixed probabilities (or are determined by a known algorithm.)
If we don't place limits on the attacker, we can never win. For example, if the attacker is all-powerful it can just peek inside the bucket. If the attacker can send an infinite number of queries per second - infinity times any small number is still infinity.
So we define the costs and limits. An attacker's cost is time and queries. They need to complete an attack before sufficient clock time (literally meaning hours or days) elapses, and they need to complete the attack using less than a finite number of queries. This number of queries is actually chosen to be a function of clock time - we assume the attacker has infinite bandwidth and is only gated by how quickly they can generate queries. We also assume the attacker is able to control the network of the server for a limited period of time - meaning they can isolate the server from the internet and ensure the only queries it receives are the attacker's. (Not that the server knows this of course.)
The defender's cost is disk space. With infinite disk space, the defender can win - we must design a mechanism that allows the defender to win without using infinite disk space.
An attacker WINS if they can achieve ANY of these three objectives:
- Determine with certainty greater than 50% whether an item remains in the opponent's bucket while sending fewer than M queries to the opponent.
- Determine with certainty greater than 50% whether an item remains in the opponent's bucket before N amount of time has past
- Cause the defender to use more than O bytes of storage.
M is chosen to be a number of queries that we consider feasible for an attacker to do in a set period of time. N is chosen to be long enough that sustaining the attack represents undue political or technical burden on an adversary. O is chosen to be a disk space size large enough that client developers or server operators are scared off of deploying Gossip.
Let's nail down M. RC4NoMore claims an average of 4450 requests per second from a javascript-driven web browser to a server. They had an incentive to get that number as high as they can, so we're going to use it. We'll pick an arbitrary amount of click time for the attacker to do this - 2 straight days. That's 768,960,000 queries or ~768 Million. Now technically, an adversary could actually perform more queries than this in a day under the situation when the 'server' is a real HTTP server, and not the client-we're-treating-as-the-server -- but we you'll see in a bit we can't provide protection against 768 Million queries, so why use a bigger number?
Those numbers are pretty well established, but what about N and O? Basically, we can only make a 'good guess' about these. For example, sustaining a BGP hijack of Twitter or Facebook's routes for more than a short period of time would be both noticeable and potentially damaging politically. TLS MITM attacks have, in the past, been confined to brief period of time. And O? How much disk space is too much? In both cases we'll have to evaluate things in terms of "I know it when I see it."
An Introduction to the Statistics We'll Need
Let's dive into the math and see, if we use the structure above, how we might design a defense that meets our 768-million mark.
It turns out, the statistics of this isn't that hard. We'll use a toy example first.
- When I query the server, it has a 10% chance of returning an object, if it has it - and it performs this 10% test for each item. (You'll note that one of the assumptions we make about the 'Retrieval Algorithm' is that is evaluates each item independently.)
Thanks to the wonder of statistics - if it never sends me the object, then is no way to be certain it does not have it. I could have just gotten really, really unlucky over those umpteen million queries.
But the probability of being that unlucky, of not receiving the object after N queries if the server has it - that can be calculated. I'll call this, colloquially, being 'confident' to a certain degree.
How many queries must I make to be 50% confident the server does not have an object? 75%? 90%?
- Assume the server has the item. The probability of not receiving the item after one query is 90%.
- After two queries: 90% x 90% or 81%. Successive multiplications yield the following:
- ~59% chance of not receiving the item after 5 queries
- ~35% chance of not receiving the item after 10 queries
The equation is a specific instance of the Binomial Probability Formula:
F(n) = nCr * p^r * q^(n-r)
nCr is the 'n choose r' equation: n! / (r! * (n-r)!)
p is the probability of the event happening (here .1)
r is the number of desired outcomes (here it is 0 - we want no item to be returned)
q is the probability of the event not happening (here 1 - .1 or .9)
n is the number of trials
Our equations can be checked:
I must make 22 queries to be 90% confident the server does not have the item.Also worth noting is that equation can be thankfully simplified. Because r is 0, we only need to calculate q^(n) - which matches our initial thought process.
Going Back to the 768 Million
So here's what to do with this math: I can use this method to figure out what the probability of sending an item will need to be, to defend against an attacker using the definition of winning we define above. I want .50 = q^(768million). That is to say, I want, after 768 Million queries, an attacker to have a 50% confidence level that the item does not remain in the bucket.
Now it just so happens that Wolfram Alpha can't solve the 768-millionth root of .5, but it can solve the 76.896 millionth root of .5 so we'll go with that. It's .99999999098591.
That is to say, to achieve the 50% confidence interval the probability of sending an item from the bucket needs to be about .00000009%.
Do you see a problem here? One problem is that I never actually defined the defender having the goal of ever sending an item! At this probability, an item has a 50% of being sent after about 50 million requests. I don't know how long it takes Google to reach the number of visits - but realistically this means the 'evidence of attack' would just never get shared.
So.... Send it more frequently?
This math, sending it so infrequently, would surely represent the end game. In the beginning, surely we would send the item more frequently, and then the more we send it, the less often we would send it. We could imagine it as a graph:
| | x | x | x | x | x | x | x | x | x | x | x | x | x | x | x | x | x +-------------------------------------------------------------------------------
But the problem, remember, is not just figuring out when to send the item, but also when to delete it.
Consider Deleting After Sending?
Let's imagine a simple deletion algorithm.
- The server will 'roll for deletion' after sending the item to a client who requests it.
- The likelihood of deletion shall be 1%.
Now recall in the beginning, after an item is newly placed into the bucket, it shall be sent with high probability. Let's fix this probability at a lowly 40%, and say this probability applies for the first 500 times it is sent. What is the probability that an item has been deleted by the 500th response? It is 99%. And how many queries are needed on average by the attacker to have the item returned 500 times at 40% probability of sending? It is (thanks to some trial and error) 1249.
What this means is that an attacker who sends on average 1249 queries in the beginning (right after the evidence of the attack goes into the bucket) can be supremely confident that the item has been deleted.
Then, the attacker sends more queries - but far fewer than the 768-million figure. If the item is not returned in short order, the attacker can be very confident that the item was deleted. This is because at the top of that curve, the likelihood of receiving the item quickly is very good. When the item doesn't appear quickly, it's either because the attacker hit a .000000001% chance of being unlucky - or it's because the item was deleted.
'Rolling for deletion' after an item is sent is a poor strategy - it doesn't work when we want to send the item regurally.
A Deletion Algorithm That May Work
- The server will 'roll for deletion' every hour, and the odds of deleting an item are... we'll say 5%.
We can use the Binomial Probability Formula, again, to calculate how likely we are to delete the item after so many hours. It's 1 minus the probability of the deletion not occurring, which is .95num_hours
- 51% chance of deletion after 14 hours.
- 71% chance of deletion after 24 hours
- 91% chance of deletion after 48 hours
If we use a rough yardstick of 'Two Days' for the attacker's timeframe (with deletion rolls once an hour) to yield a 50% confidence level, the equation becomes .50 = q^48 or a 1.4% chance of deletion.
But What About Uncertainty!
If you're following along closely, you may have realized a flaw with the notion of "1.4% chance of deletion every hour." While it's true that after 2 days the probability an item is deleted is 50%, an attacker will be able to know if it has been deleted or not!
This is because the attacker is sending tons of queries, and we already determined that trying to keep the attacker in the dark about whether an item is 'in the bucket' requires such a low probability of sending the item that it's infeasible. So the attacker will know whether or not the item is in the bucket, and there's a 50% chance (that the attacker cannot influence) of it being deleted after two days.
This not ideal. But it seems to the best tradeoff we can make. The attacker will know whether or not the evidence has been erased, but can do nothing to encourage it to be erased. They merely must wait it out.
But what About Disk Space?
So far what we've determined is:
- A deletion algorithm that is based on how often the server sends the item won't work.
- A deletion algorithm that is based on time seems like it will work...
But we haven't determined how much disk will be used by this algorithm. To calculate this number, we must look at the broader CT and CT Gossip ecosystem.
We store two types of data STHs, and [SCTs+Cert Chains]. These are stored by both a Web Browser and Web Server. STHs and SCTs are multiplied by the number of trusted logs in the ecosystem, which we'll place at '20'. We'll make the following size assumptions:
- The size of a SCT is ~120 bytes.
- The size of a STH is ~250 bytes.
- A certificate chain is 5KB.
- But a disk sector is 4KB, so everything is 4KB, except for the chain which is 8KB. (Note that this is 'naive storage'. It doesn't include any associated counters or metadata which would increase size, nor does it include more efficient storage mechanisms which would decrease size.)
A server's SCT Store will be limited by the number of certificates issued for the domains it is authoritative for multiplied by the number of logs it trusts. Let's be conservative and say 10,000 certs. ((10000 SCTs * 4 Kb * 20 logs) + (10000 Cert Chains * 8kb)) / 1024 Kb/Mb = 860MB. That's a high number but it's not impossible for a server.
A server's STH store could in theory store every active STH out there. We limit Gossip to STHs in the past week, and STHs are issued on average once an hour. This would be (20 logs * 7 days * 24 hours * 4 Kb) / 1024 Kb/Mb = 13.1MB and that's quite reasonable.
On the client side, a client's STH store would be the same: 13.1MB.
Its SCT store is another story though. First, there is no time limit for how long I may store a SCT. Secondly, I store SCTs (and cert chains) for all sites I visit. Let's say the user has visited 10000 sites, each of which have 3 different certificates with 10 SCTs each. That's ((10000 Sites * 3 Cert Chains * 8 Kb) + (10000 Sites * 3 Certificates * 10 SCTs * 4 Kb)) / 1024 Kb/Mb) / 1024 Mb/Gb = 1.4 GB. On a client, that's clearly an unacceptable amount of data.
Deleting Data From the Client
So what we want to solve is the disk-space-on-the-client problem. If we can solve that we may have a workable solution. A client whose SCT Store is filling up can do one, or more, of the following (plus other proposals I haven't enumerated):
- Delete data that's already been sent
- Delete new, incoming data (freeze the state)
- Delete the oldest data
- Delete data randomly
I argue a mix of the the first and last is the best. Let's rule out the middle two right away. These are purely deterministic behavior. If I want to 'hide' a piece of evidence, I could either send it, then fill up the cache to flush it, or flood the cache to fill it up and prevent it being added.
On its face, deleting data at random seems like a surefire recipe for failure - an attacker performs an attack (which places the evidence item in the bucket), then floods the bucket with new items. Once the bucket if full, the probability of the the evidence item being deleted rises with each new item placed in. (With a 30,0000 item cache, the odds of evicting a particular item is 50% after 51,000 queries - 30,000 queries to fill it and 21,000 to have a 50% chance of flushing it.) These numbers are far short of 768-million query figure we wish to protect ourselves against.
Deleting data that's already been sent is a good optimization, but does not solve the problem - if an attacker is flooding a cache, all of the data will be unsent.
We seem to be sunk. In fact - we were unable to come to a generic fix for this attack. The best we can do it make a few recommendations that make the attack slightly more difficult to carry out.
- Aggressively attempt Inclusion Proof Resolution for SCTs in the cache. If the SCT is resolved, discard the SCT and save the STH. If this particular SCT is not resolved, but others are, save this SCT. If all SCT resolution fails, take no special action.
- Prioritize deleting SCTs that have already been sent to the server. If a SCT has been sent to the server, it means it has been sent over a connection that excludes that SCT. If it was a legit SCT, all is well (it's been reported). If it was a malicious SCT - either it's been reported to the legitimate server (and ideally will be identified) or it's been reported to an illegitimate server necessitating a second, illegitimate SCT we have in our cache.
- In the future, it may be possible for servers to supply SCTs with Inclusion Proofs to recent STHs; this would allow clients to discard data more aggressively.
Conclusion
The final recommendation is therefore:
- Servers and Clients will each store valid STHs without bound. The size needed for this is a factor of the number of logs and validity window (which is one week). The final size is manageable, under 20MB with naive storage.
- Servers will store SCTs and Certificate Chains without bound. The size needed for this is a factor of the number of certificates issued for domains the server is authoritative for, and the number of logs. The final size is manageable for most servers (under 1GB with naive storage) and can be reduced by whitelisting certain certificates/SCTs to discard.
- Clients will store SCTs and Certificate Chains in a fixed-size cache of their choosing, employ strategies to make flushing attacks more difficult, but ultimately remain vulnerable to a persistent flushing attack.
Recently I wanted to run a complex query across every certificate in the CT logs. That would obviously take some time to process - but I was more interested in ease-of-execution than I was in making things as fast as possible. I ended up using a few tools, and writing a few tools, to make this happen.
- catlfish
- Catlfish is a CT Log server that's written by a friend (and CT Gossip coauther). I'm not interested in the log server, just the tools - specifically fetchallcerts.py to download the logs.
- fetchalllogkeys.py
- fetchallcerts.py requires the log keys, in PEM format. (Not sure why.) Run this tool to download all the logs' keys.
- update-all.sh
- fetchallcerts.py only works on one log at a time. A quick bash script will run this across all logs.
With these tools you can download all the certificates in all the logs except the two logs who use RSA instead of ECC. (That's CNNIC and Venafi.) They come down in zipfiles and take up about 145 GB.
Now we need to process them! For that you can use findcerts.py. The way this script works is using python's multiprocessing (one process per CPU) to process a zipfile at a time. It uses pyasn1 and pyx509 to parse the certificates. You write the filtering function at the top of the file, you can also choose which certs to process (leaf, intermediate(s), and root). You can limit the filtering to a single zip file (for testing) or to a single log (since logs will often contain duplicates of each other.)
The example criteria I have in there looks for a particular domain name. This is a silly criteria - there are much faster ways to look for certs matching a domain name. But if you want to search for a custom extension or combination of extensions - it makes a lot more sense. You can look at pyx509 to see what types of structures are exposed to you.
A word on minutia - pyasn1 is slow. It's full featured but it's slow. On the normal library it took about 18 minutes to process a zip file. By using cython and various other tweaks and tricks in both it and pyx509, I was able to get that down to about 4 minutes, 1.5 if you only process leaf certs. So I'd recommend using my branches of pyasn1 and pyx509.
All in all, it's definetly not the fastest way to do this - but it was the simplest. I can run a query across one of the google logs in about 18 hours, which is fast enough for my curiosity for most things.
A little bit ago NCC Group North America had an all-hands retreat, and solicited technical talks. I fired off a one-line e-mail: "All About Tor - Everything from the Directory Authorities to the Link Protocol to Pluggable Transports to everything in between." And promptly forgot about it for... a couple months. I ended up building the deck, with a level of detail I thought was about 80% of what I wanted, and gave a dry-run for my 45 minute talk. It ran two full hours.
I cut a bunch of content for the talk, but knew I would need to finish the whole thing and make it available. Which I finally did! The slides are available here, and are released CC Attribution-ShareAlike. The source for the presentation is available in keynote format.
Major thanks to all the folks I bugged to build this, especially Nick Mathewson, and those who gave me feedback on mailing lists.

required, hidden, gravatared
required, markdown enabled (help)
* item 2
* item 3
are treated like code:
if 1 * 2 < 3:
print "hello, world!"
are treated like code: